krotepi.blogg.se - Stata confidence interval

#STATA CONFIDENCE INTERVAL HOW TO#

įortunately, you can check assumptions #4, #5 and #6 using Stata. If you do not have independence of observations, it is likely you have "related groups", which means you will need to use a one-way repeated measures ANOVA instead of the one-way ANOVA. For example, there must be different participants in each group with no participant being in more than one group.

Assumption #3: You should have independence of observations, which means that there is no relationship between the observations in each group or between the groups themselves.

Examples of categorical variables include gender (e.g., 2 groups: male and female), ethnicity (e.g., 3 groups: Caucasian, African American and Hispanic), physical activity level (e.g., 4 groups: sedentary, low, moderate and high), and profession (e.g., 5 groups: surgeon, doctor, nurse, dentist, therapist).

Assumption #2: Your independent variable should consist of two or more categorical, independent (unrelated) groups.

If your dependent variable is ordinal, you might consider running a Kruskal-Wallis H test instead. If you are unsure whether your dependent variable is continuous (i.e., measured at the interval or ratio level), see our Types of Variable guide. Examples of such continuous variables include height (measured in feet and inches), temperature (measured in ☌), salary (measured in US dollars), revision time (measured in hours), intelligence (measured using IQ score), reaction time (measured in milliseconds), test performance (measured from 0 to 100), sales (measured in number of transactions per month), and so forth.

Assumption #1: Your dependent variable should be measured at the continuous level.

However, you should decide whether your study meets these assumptions before moving on. Since assumptions #1, #2 and #3 relate to your study design and choice of variables, they cannot be tested for using Stata. If any of these six assumptions are not met, you cannot analyse your data using a one-way ANOVA because you will not get a valid result. There are six "assumptions" that underpin the one-way ANOVA. However, before we introduce you to this procedure, you need to understand the different assumptions that your data must meet in order for a one-way ANOVA to give you a valid result.

#STATA CONFIDENCE INTERVAL HOW TO#

This "quick start" guide shows you how to carry out a one-way ANOVA with post hoc tests using Stata, as well as how to interpret and report the results from this test.

You need to conduct these post hoc tests because the one-way ANOVA is an omnibus test and cannot tell you which specific groups were significantly different from each other it only tells you that at least two groups were different.

When there is a statistically significant difference between the groups, it is possible to determine which specific groups were significantly different from each other using post hoc tests. Alternately, a one-way ANOVA could be used to understand whether there is a difference in salary based on degree type (i.e., your dependent variable would be "salary" and your independent variable would be "degree type", which has five groups: "business studies", "psychology", "biological sciences", "engineering" and "law"). Alternatively, if you have multiple dependent variables you can consider a one-way MANOVA.įor example, you can use a one-way ANOVA to determine whether exam performance differed based on test anxiety levels amongst students (i.e., your dependent variable would be "exam performance", measured from 0-100, and your independent variable would be "test anxiety levels", which has three groups: "low stressed students", "medium stressed students, and "high stressed students"). If you have two independent variables you can use a two-way ANOVA. However, it is typically only used when you have three or more independent, unrelated groups, since an independent-samples t-test is more commonly used when you have just two groups.

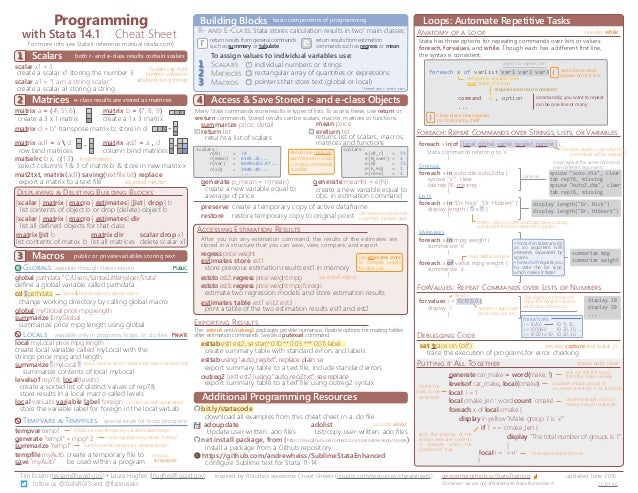
The one-way analysis of variance (ANOVA) is used to determine whether the mean of a dependent variable is the same in two or more unrelated, independent groups.